Intervals, Major Triads, and Inversions

In this lesson, we will learn about major triads. In previous lessons, you learned how to construct a major scale. Using a few simple techniques, I’ll show you how to easily transform a major scale into a major triad, when you’re most likely to play major triads, and some common major triad progressions.
What is a Chord?
A chord can be described by the following characteristics:
- A set of notes played together to produce a specific type of sound.
By definition, a chord is a combination of pitches that harmonise when sounded together. The simplest chords consist of three notes, sometimes called triads.
To reiterate:
- A chord with 3 notes is called a triad.
- A chord with 4 notes is called a seventh chord.
- A chord with 5 notes is called a ninth chord.
- A chord with 6 notes is called an eleventh chord.
- A chord with 7 notes is called a thirteenth chord.
| Number of Notes | Chord Type |
|---|---|
| 3 | Triad |
| 4 | Seventh Chord |
| 5 | Ninth Chord |
| 6 | Eleventh Chord |
| 7 | Thirteenth Chord |
Intervals
In music, an interval is the pitch distance between two notes. Intervals are calculated from the lower note to the higher note, with the lower note counted as 1.
Intervals are named by the numerical position of the upper note (2nd, 3rd, etc.), with two exceptions:
- The interval between two identical notes is called a unison (also known as a perfect prime).
- The interval spanning eight scale degrees is called an octave.
Perfect Intervals and Major Intervals
An interval from the tonic of a major scale to its unison, 4th, 5th, or 8th is called a perfect interval.
- Example: In the C major scale, the distance from C to G is a perfect fifth. From C to F is a perfect fourth. The 8th note of the scale is a perfect octave, and the distance between identical notes is a perfect unison.
default:
Piano.Keyboard:
showKeyName: true
children: C,F
first: C0
last: B0
label: "Perfect fourth"
activeColor: [null, burlywood]
default:
Piano.Keyboard:
showKeyName: true
children: C,G
first: C0
last: B0
label: "Perfect Fifth"
activeColor: [null, burlywood]
Intervals between the tonic and the 2nd, 3rd, 6th, or 7th degrees of a major scale are called major intervals.
- Example: In the C major scale, C to D is a major second, C to E is a major third, C to A is a major sixth, and C to B is a major seventh.
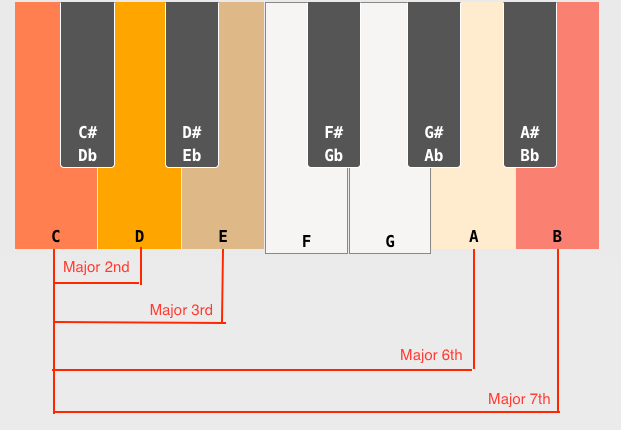
Minor Intervals
When a major interval (2nd, 3rd, 6th, or 7th) is reduced by a half step, it becomes a minor interval.

- Example: A major third (C to E) becomes a minor third (C to E♭). A minor second is D♭ instead of D, a minor sixth is A♭ instead of A, and a minor seventh includes B♭ instead of B.
- Note: Only major intervals can become minor—perfect intervals cannot (e.g., there’s no "minor perfect fourth").
Melodic vs. Harmonic Intervals
- Melodic intervals: Played separately (e.g., in a melody).
- Harmonic intervals: Played together (e.g., in a chord).
- Since we’re studying chords, we focus on harmonic intervals.
Key Insight:
- A major triad = major third + perfect fifth.
- A minor triad = minor third + perfect fifth.
Augmented and Diminished Intervals
- Augmented: A perfect or major interval expanded by a half step.
- Example: A perfect fifth (C to G) becomes an augmented fifth (C to G♯).
- Diminished: A perfect or minor interval reduced by a half step.
- Example: A perfect fifth (C to G) becomes a diminished fifth (C to G♭).
Chromatic Intervals
An interval is chromatic if its notes do not belong to the same major scale.
- In major scales, minor, diminished, and augmented intervals are always chromatic.
Major Triads
A major triad is a three-note chord built on the 1st, 4th, or 5th degrees of a major scale. These are labeled with Roman numerals I (1), IV (4), V (5).
default:
Piano.Chart:
showKeyName: true
children: CM, FM, GM
first: C0
last: B1
label: "C triads, F triads, G triads"
- Example: In C major:
- C major triad (I): C-E-G
- F major triad (IV): F-A-C
- G major triad (V): G-B-D
Why major scales matter:
- Without knowing the C major scale, how would you know E is the major third of C or G is the perfect fifth?
Two Ways to Build a Major Triad
- Using the major scale:
- Take the 1st, 3rd, and 5th notes of the scale.
- Example: In F major, F-A-C = F major triad.
- Stacking intervals:
- Major third (4 semitones) + minor third (3 semitones).
- Example: For a G major triad:
- Start with G (root).
- Add a major third (G to B).
- Add a minor third above B (B to D).
Inversions
A triad (3-note chord) can be played in three positions:
- Root position: Root is the lowest note (e.g., C-E-G).
- First inversion: Third is the lowest note (e.g., E-G-C).
- Second inversion: Fifth is the lowest note (e.g., G-C-E).
Extended chords (7th, 9th, etc.) have more inversions. For example:
- A 7th chord (4 notes) has third inversion (7th as the lowest note).
Example: C Major Triad Inversions
- Root position: C-E-G
- First inversion: E-G-C
- Second inversion: G-C-E





